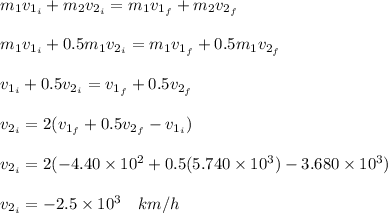Answer:
Speed of comet before collision is
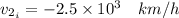
Step-by-step explanation:
Correction: (As stated after collision comet moves away from moon so velocity of moon and moon and comet must be opposite in direction. as spped of moon after collision is −4.40 × 10^2km/h so that comet's must be 5.740 × 10^3km/h instead of -5.740 × 10^3km/h)
Solution:
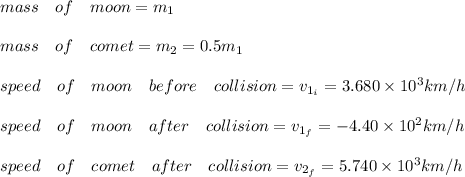
Case is considered as partially inelastic collision, by conservation of momentum