Question

The student population at the University is currently 400. The expected annual rate of increase is predicted to be 0.02. What will the population be in five years? Round your answer to the nearest whole number.

Answer 1

The population of the students at the University after 5 years is 442.

Explanation:

Given:

Current population of students is,
`P_o=400`

Growth rate is,
`r=0.02`

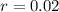
Time after which population is needed is,
`t=5\ years`

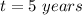
Let 'P' be the population after 't' years.

Population growth is an exponential growth and the equation to determine the population after 't' years is given as:

`P=P_oe^(rt)`

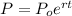
Now, plug in 400 for
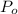`P_o`, 0.02 for 'r', 5 for 't' and solve for 'P'. This gives,

`P=(400)e^(0.02* 5)\\\\P=400* e^(0.1)\\\\P=400* 1.1052\\\\P=442`

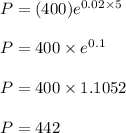
Therefore, the population of the students at the University after 5 years is 442.