The equation of line in standard form is 5x - 4y = -38
Solution:
Given that we have to find the equation of line perpendicular to 4x + 5y = 40 that includes the point (-10, -3)
The equation of line in slope intercept form is given as:
y = mx + c ------ eqn 1
Where "m" is the slope of line and "c" is the y - intercept
Given equation of line is:
4x + 5y = 40
Rearranging to slope intercept form, we get
5y = -4x + 40

On comparing the above equation with eqn 1,

We know that product of slope of line and slope of line perpendicular to given line is equal to -1
Therefore,

Now find the equation of line with slope 5/4 and passing through (-10, -3)
Substitute
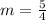 and (x, y) = (-10, -3) in eqn 1
and (x, y) = (-10, -3) in eqn 1
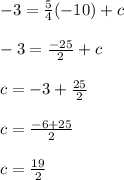
Substitute
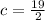 and
and
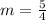 in eqn 1
in eqn 1

The standard form of an equation is Ax + By = C
Therefore,

Thus the equation of line in standard form is found