Question

Through: (-5, 5), perp. to y = 5/9x - 4

Answer 1

`y=-(9)/(5)x-4`

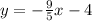
Explanation:

The complete question is:

Find the equation of the line that passes through: (-5, 5), and is perpendicular to the line y = 5/9x - 4

step 1

Find the slope of the line

we know that

If two lines are perpendicular, then their slopes are opposite reciprocal (the product of their slopes is equal to -1)

The equation of the given line is

`y=(5)/(9)x-4`

The slope of the given line is

`m_1=(5)/(9)`

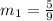
The opposite reciprocal is equal to

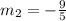`m_2=-(9)/(5)` ----> slope of the perpendicular line

step 2

Find the equation of the line in point slope form

`y-y1=m(x-x1)`

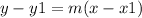
we have

`m=-(9)/(5)`

`point\ (-5,5)`

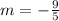
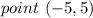
substitute

`y-5=-(9)/(5)(x+5)`

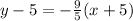
step 3

Convert to slope intercept form

Isolate the variable y

`y-5=-(9)/(5)(x+5)\\\\y-5=-(9)/(5)x-9\\\\y=-(9)/(5)x-9+5\\\\y=-(9)/(5)x-4`