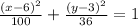Answer:
The equation of a vertical ellipse is
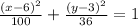
Explanation:
Here, given:
Length of major axis: 20
⇒ 2 a = 20 , or , a = 10
Length of minor axis: 12
⇒ 2 b = 12 , or , b = 6
Also, center (h,k) = (6,3)
Now, STANDARD EQUATION OF ELLIPSE :
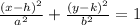
Now, substituting the values, a, b , h and k in above expression, we get:
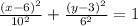
or,
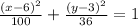
Hence, the equation of a vertical ellipse is