Question

In previous​ tests, baseballs were dropped 24 feet onto a concrete​ surface, and they bounced an average of 92.82 inches. In a test of a sample of 23 new​ balls, the bounce heights had a mean of 92.6 inches and a standard deviation of 1.72 inches. Use a 0.05 significance level to determine whether there is sufficient evidence to support the claim that the new balls have bounce heights with a mean different from 92.8292.82 inches. Does it appear that the new baseballs are​ different?

Answer 1

There is sufficient evidence to support the claim that the new balls have bounce heights with a mean different from 92.8292.82 inches, and it appears that the new baseballs are​ different

Explanation:

Given that in previous​ tests, baseballs were dropped 24 feet onto a concrete​ surface, and they bounced an average of 92.82 inches

But new balls showed mean of 92.6 inches with s = 1.72 inches

Sample size = 23

Since sample size is less than 30 and population std deviation is not know we use t test

`H_0: \bar x = 92.82\\H_a: \bar x \\eq 92.82`

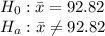
(Two tailed test at 5% significance level)

Mean difference =
`92.6-92.82=-1.22`

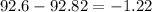
Std error of sample mean = s/sqrt n =
`(1.72)/(√(23) ) \\=0.3586`

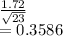
Test statistic t = mean diff/std error = -3.402

df = 23-1 =22

p value = 0.002559

since p value <5% we reject H0

There is sufficient evidence to support the claim that the new balls have bounce heights with a mean different from 92.8292.82 inches, and it appears that the new baseballs are​ different