Question

in the diagram below of triangle NPQ, R is a midpoint of NP and S is a midpoint of PQ. If RS = 47 - 5x, and NQ = 5x - 11, what is the measure of RS?​

Answer 1

Therefore the measure of RS is 12 unit.

Explanation:

Given:

In ΔNPQ,

R is a midpoint of NP and

S is a midpoint of PQ.

RS = 47 - 5x, and NQ = 5x - 11

To Find:

RS = ?

Solution:

Mid Point Theorem:

The line segment joining the midpoints of two sides of a triangle is parallel to the third side and is equal to one half of the third side.

Here ,R is a midpoint of NP and

S is a midpoint of PQ.

∴
`RS=(1)/(2)NQ`

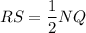
Substituting the values we get

`47-5x=(1)/(2)(5x-11)\\\\2(47-5x)=5x-11\\\\94-10x=5x-11\\5x+10x=94+11\\15x=105\\x=(105)/(15)=7\\x=7`

Now Substituting ' x ' in RS we get

`RS=47-5* 7=47-35=12\\RS=12\ unit`

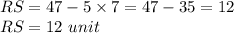
Therefore the measure of RS is 12 unit.