Answer:
It is clear that, The Shelly account is $11.23 more than that in Anne account .
Explanation:
Given as :
For Anne
The amount deposited in account = p = $500
The rate of interest = r = 6% at simple interest
The time period of deposit = t = 4 years
Let The amount received in account after 4 years = $

From Simple Interest method
Simple Interest =
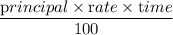
Or, s.i =

Or, s.i =
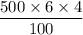
Or, s.i =
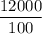
i.e s.i = $120
∵ Amount = Principal + Interest
Or,
 = p + s.i
= p + s.i
Or
 = $500 + $120
= $500 + $120
Or, Amount = $620
So, The Amount in Anne account after 4 years is $620
Again
For, Shelly
The amount deposited in account = P = $500
The rate of interest = R = 6% compounded annually
The time period of deposit = T = 4 years
Let The amount received in account after 4 years = $

From Compound Interest method
Amount = Principal ×
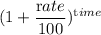
Or,
 = P ×
= P ×
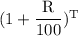
Or,
 = $500 ×
= $500 ×
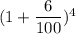
Or,
 = $500 ×
= $500 ×

Or,
 = $500 × 1.26247
= $500 × 1.26247
Or,
 = $631.23
= $631.23
So, The amount received by Shelly in her account after 4 years = $631.23
Now, Difference between amount received in their account
i.e
 -
-
 = $631.23 - $620
= $631.23 - $620
Or,
 -
-
 = $11.23
= $11.23
Hence, It is clear that, The Shelly account is $11.23 more than that in Anne account . Answer