To develop this problem we will apply the Archimedes model. As well as the definitions of Weight based on mass and acceleration. The first in turn will be considered under the relationship of Density and Volume. From the values given we have to:
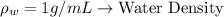
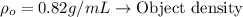
Since it is in equilibrium, the weight of the object will have a reaction from the water, which will cause the sum of forces between the two objects to be zero, therefore
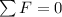
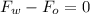
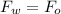
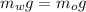
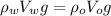
The value of gravity is canceled because it is a constant
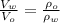

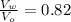
The portion of the object that is submerged corresponds to 82%, while the portion that is visible, above the water level will be 18%