Question

Determine whether the relationship between the two quantities is proportional. Explain.

A. No, because the graph of the line connecting the ordered pairs passes through the origin. 

B. No, because the graph of the line connecting the ordered pairs does no pass through the origin. 

C. Yes, because the graph of the line connecting the ordered pairs passes through the origin. 

D. Yes, because the graph of the line connecting the ordered pairs does not pass through the origin.

Answer 1

Option B.

Explanation:

Answer 2

Option B. No, because the graph of the line connecting the ordered pairs does no pass through the origin.

Explanation:

we know that

A relationship between two variables, x, and y, represent a proportional variation if it can be expressed in the form
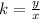`k=(y)/(x)` or
`y=kx`

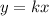
In a proportional relationship the constant of proportionality k is equal to the slope m of the line and the line passes through the origin

step 1

Connect the ordered pairs of the graph

The y-intercept is the point (0,20)

see the attached figure

That means, that the line not passes through the origin

therefore

The relationship between the two quantities is not proportional