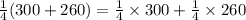Question

At a ski resort, 1/4 of the 300 skiers are beginners, and 1/4 of the 260 snowboarders are beginners. The resort staff used the distributive property to make the calculation of the total number of beginners on the ski slopes. Write an expression demonstrates the distributive property?

16 points

Answer 1

To demonstrate the distributive property, the expression (1/4) ×(300 + 260) can be used to calculate the total number of beginners on the ski slopes, which is equal to 140 beginners.

Step-by-step explanation:

To write an expression that demonstrates the distributive property, we can use the information given in the question:

1/4 of the 300 skiers are beginners, which is equal to (1/4) × 300 = 75 beginners.

1/4 of the 260 snowboarders are beginners, which is equal to (1/4) × 260 = 65 beginners.

To calculate the total number of beginners on the ski slopes, we can use the distributive property:

Total beginners = (1/4) × (300 + 260) = (1/4) × 560 = 140 beginners.

Answer 2

`(1)/(4)(300 + 260) = (1)/(4) * 300 + (1)/(4) * 260` is the expression that demonstrates the distributive property

Solution:

Given that,

At a ski resort,
`(1)/(4)` of the 300 skiers are beginners

`(1)/(4)` of the 260 snowboarders are beginners

The resort staff used the distributive property to make the calculation of the total number of beginners on the ski slopes

Beginners in skiers =
`(1)/(4)` of the 300

Beginners in snowboarders =
`(1)/(4)` of the 260

Total number of beginners = Beginners in skiers + Beginners in snowboarders

`\text{ Total number of beginners } = (1)/(4)(300) + (1)/(4)(260)`

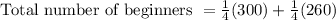
We have to use distributive property

The distributive property of multiplication states that when a number is multiplied by the sum of two numbers, the first number can be distributed to both of those numbers and multiplied by each of them separately, then adding the two products together for the same result as multiplying the first number by the sum

a(b + c) = ab + bc

Therefore, our expression is:

`(1)/(4)(300) + (1)/(4)(260)`

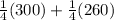
We have to take
`(1)/(4)` as common term to get distributive property

`(1)/(4)(300) + (1)/(4)(260) = (1)/(4)(300 + 260)\\\\(1)/(4) * 300 + (1)/(4) * 260 = (1)/(4)(300 + 260)`

Thus distributive property is applied