Answer:
The radius of this circle is
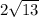 and the central angle of the sector is
and the central angle of the sector is
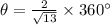 .
.
Explanation:
Consider the provided information.
If a conical paper funnel is made out of a sector of circle then the radius of the sector becomes the slant height of the cone, and the length of the curved part of the sector becomes the circumference of the base of the cone.
First find the slant height:
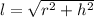
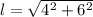
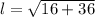
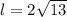
Thus, the radius of the sector is
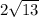 which is same as the slant height of the cone.
which is same as the slant height of the cone.
Now, the circumference of the base of the cone is same as the length of the curved part.
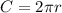
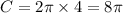
The length of the curved part =
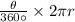
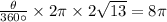

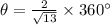
Hence, the radius of this circle is
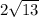 and the central angle of the sector is
and the central angle of the sector is
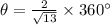 .
.