Question

A writing workshop enrolls novelist and poets in a ratio of 5:3. There are 24 people at the workshop. How many novelist are there? How many poets are there?

Answer 1

There are 15 novelist and 9 poets at the writing workshop.

Explanation:

Given,

Total number of people at the workshop = 24

We have to find the number of novelist and poets at the workshop.

Solution,

Since the ratio of novelist and poets is 5:3.

Then we can say that the number of novelist is '5x' and the number of poets is '3x'.

Now according to question, total number of people is the sum of number of novelist and number of poets.

So, framing the above sentence in equation form, we get;

Total number of people = number of novelist + number of poets

On substituting the given values, we get;

`5x+3x=24\\\\8x=24`

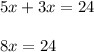
On using division property we will divide by '8' on both side and get;

`(8x)/(8)=(24)/(8)\\\\x=3`

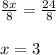
Now we substitute the value of 'x' to get the number of novelist as well as poets.

Number of novelist =
`5x=5*3=15`

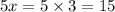
Number of poets =
`3x=3*3=9`

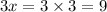
Hence There are 15 novelist and 9 poets at the writing workshop.