Question

NEED HELP ASAP!!!

Write the equation of the perpendicular bisector of \overline{AB} A B ¯ if A(–6, –4) and B(2, 0).
Group of answer choices
y=-2x-2 y = − 2 x − 2
This answer is set as correct T h i s a n s w e r i s s e t a s c o r r e c t
y=\frac{1}{2}x-6 y = 1 2 x − 6
y=\frac{1}{2}x-2
There is a picture below of the question

Answer 1

The equation of perpendicular bisector of A(-6, -4 ) and B(2, 0) is y = -2x - 6

Solution:

Given that we have to find the equation of perpendicular bisector of A(-6, -4 ) and B(2, 0)

A perpendicular bisector, bisects a line segment at right angles

To obtain the equation we require slope and a point on it

Find the midpoint and slope of the given points and then we can find the equation

Find the midpoint:

Given points are A(-6, -4 ) and B(2, 0)

The midpoint is given as:

`m(x, y)=\left((x_(1)+x_(2))/(2), (y_(1)+y_(2))/(2)\right)`

`\text {Here } x_(1)=-6 ; y_(1)=-4 ; x_(2)=2 ; y_(2)=0`

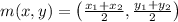
Substituting the values we get,

`\begin{aligned}&m(x, y)=\left((-6+2)/(2), (-4+0)/(2)\right)\\\\&m(x, y)=(-2,-2)\end{aligned}`

Find the slope of given points:

`m=(y_(2)-y_(1))/(x_(2)-x_(1))`

`m=(0-(-4))/(2-(-6))\\\\m = (4)/(8)\\\\m = (1)/(2)`

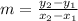
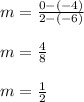
Then the slope of perpendicular bisector is given as:

We know that product of slopes of given line and slope of line perpendicular to it is equal to -1

Let the slope of perpendicular bisector be
`m_1`

`(1)/(2) * m_1 = -1\\\\m_1 = -2`

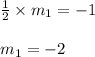
Find the equation of line with slope -2 and point (-2, -2)

The equation of line in slope intercept form is given as:

y = mx + c -------- eqn 1

Where "m" is the slope and "c" is the y - intercept

Substitute (x, y) = (-2, -2) and slope m = -2 in eqn 1

-2 = -2(-2) + c

-2 = 4 + c

c = -2 - 4

c = -6

Substitute c = -6 and m = -2 in eqn 1

y = -2x - 6

Thus the required equation of perpendicular bisector is found