Question

The number of tornadoes in the Midwest region in a year is modeled as a Poisson random variable with mean 3. Calculate the probability that at least 2 tornados occurred in the period from January 1 to June 30.

Answer 1

There is a 44.22% probability that at least 2 tornados occurred in the period from January 1 to June 30.

Explanation:

In a Poisson distribution, the probability that X represents the number of successes of a random variable is given by the following formula:

`P(X = x) = (e^(-\mu)*\mu^(x))/((x)!)`

In which

x is the number of sucesses

e = 2.71828 is the Euler number

`\mu` is the mean in the given time interval.

In this problem, we have that:

In a year, there tends to be 3 tornados. From January 1 to June 30, it is half a year. So
`\mu = 1.5`

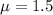
Calculate the probability that at least 2 tornados occurred in the period from January 1 to June 30.

Either there were less than two tornados on this interval, or there were two or more. The sum of the probabilities of these events is decimal 1. So:

`P(X < 2) + P(X \geq 2) = 1`

`P(X \geq 2) = 1 - P(X < 2)`

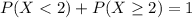
In which

`P(X < 2) = P(X = 0) + P(X = 1)`

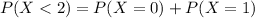
So

`P(X = x) = (e^(-\mu)*\mu^(x))/((x)!)`

`P(X = 0) = (e^(-1.5)*(1.5)^(0))/((0)!) = 0.2231`

`P(X = 1) = (e^(-1.5)*(1.5)^(1))/((1)!) = 0.3347`

`P(X < 2) = P(X = 0) + P(X = 1) = 0.2231 + 0.3347 = 0.5578`

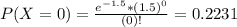
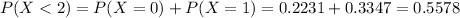
Finally

`P(X \geq 2) = 1 - P(X < 2) = 1 - 0.5578 = 0.4422`

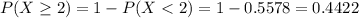
There is a 44.22% probability that at least 2 tornados occurred in the period from January 1 to June 30.