Answer : The mass of the oxygen gas produced in grams and the pressure exerted by the gas against the container walls is, 96 grams and 1.78 atm respectively.
Explanation : Given,
Moles of
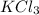 = 2.0 moles
= 2.0 moles
Molar mass of
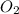 = 32 g/mole
= 32 g/mole
Now we have to calculate the moles of
The balanced chemical reaction is,
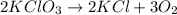
From the balanced reaction we conclude that
As, 2 mole of
 react to give 3 mole of
react to give 3 mole of
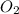
So, 2.0 moles of
 react to give
react to give
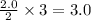 moles of
moles of
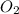
Now we have to calculate the mass of
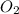
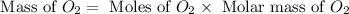

Therefore, the mass of oxygen gas produced is, 96 grams.
Now we have to determine the pressure exerted by the gas against the container walls.
Using ideal gas equation:
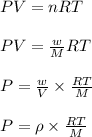
where,
P = pressure of oxygen gas = ?
V = volume of oxygen gas
T = temperature of oxygen gas =
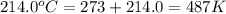
R = gas constant = 0.0821 L.atm/mole.K
w = mass of oxygen gas
 = density of oxygen gas = 1.429 g/L
= density of oxygen gas = 1.429 g/L
M = molar mass of oxygen gas = 32 g/mole
Now put all the given values in the ideal gas equation, we get:

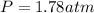
Thus, the pressure exerted by the gas against the container walls is, 1.78 atm.