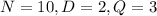Question

Jennifer has 15 coins worth a total of $1.45 all the coins are nickels dimes or quarters the number of quarters is 1/4 the number of nickels and dimes combined. Solve a linear system to find how many of each kind of coin she has?

Answer 1

`N = 10, D= 2, Q=3`

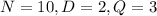
Explanation:

Notation

N= represent the number of nickels

D= represent the number of dimes

Q= represent the number of quarters

Solution to the problem

For this case we assume that a nickels represent 5 cents, a dime 10 cents and a quarter 25 cents. We know that the total ofor the 15 coins it's 1.45 or 145 cents. So we can set up the following equations:

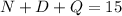`N+D+Q= 15` (1)

`5N +10D + 25Q = 145` (2)

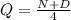`Q= (N+D)/(4)` (3)

The condition (3) is obtained from the statement "the number of quarters is 1/4 the number of nickels and dimes combined"

So on this case we have a linear system of 3 equation with 3 values unknown so then this system can be solved.

We can use substitution in order to solve the values.

We can rewrite the quation (3) on this way
`4Q= N+D`

And if we replace this into equation (1) we got:

`4Q + Q = 15`

`Q =3`

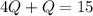
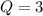
And know we can use the following two equations:

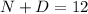`N+D = 12` (1)

`5N + 10D = 70` (2)

If we solve N from equation (1) we got:

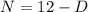`N = 12-D` and if we replace this into equation (2) we got:

`5(12-D) + 10 D = 70`

`60 -5D + 10D = 70`

`5D = 10`

`D= 2`

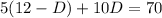
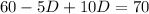
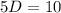
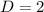
And then we can solve for N like this:

`N = 12-D= 12-2 = 10`

So then our final solution would be:

`N = 10, D= 2, Q=3`