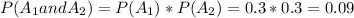Question

If two students from this college are selected at random, what is the probability that they are both males?

Answer 1

`P(A_1 and A_2) = P(A_1) *P(A_2)= 0.3*0.3 =0.09`

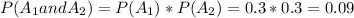
Explanation:

Assuming this problem :"Only 30% of the students in a certain liberal arts college are males. If two students from this college are selected at random, what is the probability that they are both males?"

Previous concepts

An independent event is an "event that has no connection to another event's chances of happening ". For this case we can assume that if one person is male and if we select another one the probability that this one would be male or female is totally indepedent from the first selection.

When we have two independent events let's say A and B and we want to find the probability that both events occurs at the same time we can use the following formula:

`P(A and B) = P(A)*P(B)`

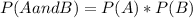
Solution to the problem

We can define some notation:

`A_1` first person selected is a male

`A_2` second person selected is male

On this case we want the probability that both would be males. And we can express this like this on math terms:

`P(A_1 and A_2)`

For this case we can assume that the two events are independent. And in order to find the probability for two events independents events we just need to multiply the probabilities of each one like this:

`P(A_1 and A_2) = P(A_1) *P(A_2)= 0.3*0.3 =0.09`