Answer:
First option 5%
Explanation:
Normal Distribution
Gauss Distribution is one of the most widely-used continuous probability distribution to characterize random events. The curve of the function is called the bell curve.
The standard Normal Distribution is a special case where the mean
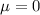 and the standard deviation
and the standard deviation
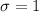 . The values of the cumulative normal distribution cannot be computed by simple calculations, we must use tables of digital implementations integrated with tools like Excel or any online resource.
. The values of the cumulative normal distribution cannot be computed by simple calculations, we must use tables of digital implementations integrated with tools like Excel or any online resource.
The entry for standard normal distribution is a parameter called the z-score, computed like shown below

The values provided in our problem are
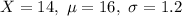
Computing z results
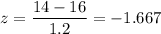
By using any of the aforementioned resources, we compute the left-tail probability
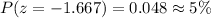
We choose the first option 5%