Answer:
22920 years
Step-by-step explanation:
half- life : Time in which sample becomes half of its original value.
It is calculated by :
n = t /half-life
Age of the sample = n x (half - life)
here, n = number of half - life
4 ----> 2 ----> 1 ----> 0.5 ---->0.25 (divide the mass each time by 1/2 )
This is equal to 4 - half life
= 4 (5730)
=22920 years
SECOND METHOD
The following equation gives relation between the original Number of species at Zero time (No) and number of species after decays (N) in time t
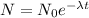
It is asked to calculate 't'.
 = Decay constant
= Decay constant
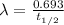
t1/2 = Half life = 5730 years
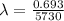
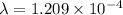 per year
per year
N= 0.25 g
No = 4.0 g
Insert the parameter in the formula and solve for t
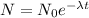
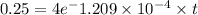
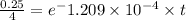

take ln(natural logarithm) both side,


= 22932.9 years (approx to 22920)