Question

Please help!! I have tried it on my own and I am stuck

1. A person noted that the angle of elevation to the top of a tree was 65 degrees at a distance of 11 feet from the tree. Using the diagram approximate the height of the tree.

5.128 feet

26.005 feet

12.14 feet

23.590 feet
2. Determine the pitch (i.e. angle) of the ram of a NASCAR turn.
40.0 degrees

32.9 degrees

57.1 degrees

29.6 degrees

Answer 1

1. 23.59 feet

2. 32.9°

Explanation:

1. See the diagram attached.

The man is 11 feet distance from the bottom of the tree.

And the angle of elevation from the man to the top of the tree is 65°.

Now, we need to approximate the height of the tree,

Using the trigonometry, we can write

`\tan 65^(\circ) = \frac{\textrm {Height of the Tree}}{\textrm {Base Distance}} = (h)/(11)`

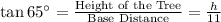
⇒ h = 11 tan 65° = 23.59 feet (Approx.) (Answer)

2. See the diagram attached.

From the given right triangle the hypotenuse is 25 feet and the base is 21 feet.

And the angle between the hypotenuse and the base is the pitch of the ram of a NASCAR turn.

Using trigonometry, we can write

`\cos \theta = \frac{\textrm {Base}}{\textrm {Hypotenuse}} = (21)/(25)`

⇒
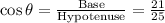
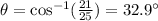`\theta = \cos^(-1)((21)/(25)) = 32.9^(\circ)` (Answer)