Question

What is the acceleration when an object traveling at 4.7 m/s accelerates to 23.9 m/s over a distance of 300 m?

Answer 1

The acceleration is approximately
`\(0.9152 \, \text{m/s}^2\)`.

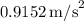Step-by-step explanation:

Acceleration is a vector quantity that describes the rate at which an object changes its velocity. It can involve changes in the object's speed, direction, or both

To calculate acceleration
`(\(a\))`, you can use the following kinematic equation:

`\[v^2 = u^2 + 2as\]`

where:

-
`\(v\)` is the final velocity,

-
`\(u\)` is the initial velocity,

-
`\(a\)` is the acceleration,

-
`\(s\)` is the displacement (change in position).

We have:

-
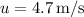`\(u = 4.7 \, \text{m/s}\)` (initial velocity),

-
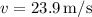`\(v = 23.9 \, \text{m/s}\)` (final velocity),

-
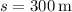`\(s = 300 \, \text{m}\)` (displacement).

Plug these values into the formula and solve for
`\(a\)`:

`\[23.9^2 = 4.7^2 + 2a(300)\]`

`\[571.21 = 22.09 + 600a\]`

`\[600a = 549.12\]`

`\[a = (549.12)/(600)\]`

`\[a \approx 0.9152 \, \text{m/s}^2\]`

So, the acceleration is approximately
`\(0.9152 \, \text{m/s}^2`\).

Answer 2

67.2 m

Explanation: source - trust me bro