Answer: The wavelength changes by a factor of

Step-by-step explanation:
The equation of the speed
 of a wave is:
of a wave is:
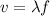
Where:
 is the speed, that remains unchanged
is the speed, that remains unchanged
 is the wavelength
is the wavelength
 is the frequency
is the frequency
Isolating
 we have:
we have:
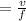
Now, if we increase the frequency by a factor of 2:
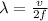
 This means the wavelength changes by a factor of
This means the wavelength changes by a factor of
