Question

Evan is working two summer jobs, making $10 per hour babysitting and making $8 per hour walking dogs. In a given week, he can work no more than 16 total hours and must earn no less than $140. If Evan worked 12 hours babysitting, determine all possible values for the number of whole hours walking dogs that he must work to meet his requirements

Answer 1

Minimum whole hour is 3 hours. Other possible values are 4 hours.

Explanation:

Given: Evan is paid for babysitting is $10 per hour.

Paid for walking dog is $8 per hour.

Maximum hour, he can work is 16 hours.

Minimum earning Evan should get in a week is $140.

Evan have worked for 12 hours as babysitting.

Now, finding minimum number of hours Evan shoud work as walking dogs to meet his goal.

As given Evan have already worked for 12 hours as babysitting, therefore lets find the amount, he earned for babysitting.

Evan´s earning in 12 hours of babysitting=
`12\ hours* \$ 10= \$ 120`

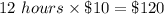
∴ Evan´s total earning in 12 hours of babysitting is $120.

Next, Hours remaining from his maximum working hours and amount to be earned to earn more to achieve his minimum earning.

Hours remaining=
`16\ hours- 12\ hours= 4\ hours`

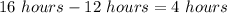
∴ 4 hours remaining after he have worked for babysitting.

Amount to be earned to achieve minimum goal=
`\$ 140-\$ 120= \$ 20`

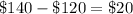
∴ Amount to be earned to achieve minimum goal on the week is $20.

If Evan work for 2 hours, then he can earn=
`2 \ hours * \$ 8= \$ 16`

$16 will not fulfill the minimum earning criteria of $140.

However, if Evan work for 3 hours, then he can earn=
`3 \ hours * \$ 8= \$ 24`

∴ Minimum 3 hours Evan have to work for walking dogs to get minimum earning of the week.

Evan can work for 4 hours also for walking dogs to get additional earning. As his Total working hour will be
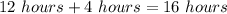`12\ hours + 4\ hours= 16\ hours` only, which is fulfilling the criteria of maximum 16 hours.