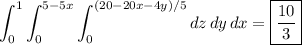You can find the intercepts of the plane with the three axes:
y = z = 0 ⇒ 20x = 20 ⇒ x = 1
x = z = 0 ⇒ 4y = 20 ⇒ y = 5
x = y = 0 ⇒ 5z = 20 ⇒ z = 4
which is to say, the plane has intercepts (1, 0, 0), (0, 5, 0), and (0, 0, 4).
For any point in the region, the z-coordinate will be bounded below by the plane z = 0 and above by the given plane z = (20 - 20x - 4y)/5.
Now shift the focus to the "shadow" of the given plane in the x-y plane. It's a triangular region in which the y-coordinate of any point in the "shadow" is bounded below by the line y = 0 and above by the line y = (20 - 20x)/4 = 5 - 5x.
Lastly, the x-coordinate is simply bounded between 0 and 1.
So, the volume of this region is