Question

Liquid helium is stored at its boiling-point temperature of4.2

K in a spherical container (r = 0.404 m). Thecontainer is
a perfect blackbody radiator. The container issurrounded by a
spherical shield whose temperature is 86.8 K. Avacuum exists in the
space between the container and shield. Thelatent heat of
vaporization for helium is 2.1 x 104J/kg. What mass of
liquid helium boils away through the ventingvalve in 15.5
hours?

Answer 1

The mass of liquid helium boiled is 8.55Kg.

Step-by-step explanation:

The heat transfer between the liquid helium spherical container and the spherical shield is made by pure radiation (there is no physical body to make the heat transfer).

Therefore we use the Stefan–Boltzmann law:

`\dot Q=\varepsilon \sigma (T_o^4-T_i^4)` (1)

But in a boiling process, the temperature of the fluid keeps constant. Therefore in al the process
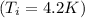`(T_i=4.2K)` (the shield temperature is constant by hypothesis).

The boiling process implies a change of phase. The heat equation in this case is:

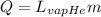`Q=L_(vapHe)m` (2)

From equation (1):

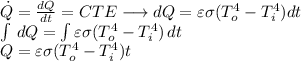`\dot Q=(dQ)/(dt) =CTE\longrightarrow dQ=\varepsilon \sigma (T_o^4-T_i^4)dt\\\int\limits \, dQ=\int\limits {\varepsilon \sigma (T_o^4-T_i^4)} \, dt\\Q=\varepsilon \sigma (T_o^4-T_i^4)t` (3)

Using equation (2) and (3):

`L_(vapHe)m=\varepsilon \sigma (T_o^4-T_i^4)t\\m=(\varepsilon \sigma (T_o^4-T_i^4)t)/(L_(vapHe))`

Because the spherical container is considered as a perfect blackbody radiator, ε=1. All the other variables are known.