Answer:
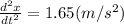 .
.
Step-by-step explanation:
To solve this problem we are going to consider the particle to be moving along the x axis and we are also going use the differential equation of the simple harmonic oscillator:
 ,
,
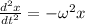 ,
,
where
 is the displacement along the x axis from the equilibrium point, and
is the displacement along the x axis from the equilibrium point, and
 is the angular frecuency.
is the angular frecuency.
We know that
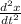 is the acceleration of the particle and that the angular frecuancy depends on the period:
is the acceleration of the particle and that the angular frecuancy depends on the period:
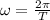 .
.
By substitution we get
 .
.
We get the maximum and minimum acceleration at a displacement equals the
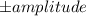 . Since we are moving along the x-axis, we get the maximum acceleration at
. Since we are moving along the x-axis, we get the maximum acceleration at
 .
.
So
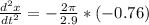 ,
,
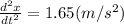 .
.