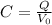Question

A parallel plate capacitor is made of two large plates ofarea

A each. One of the plates is grounded and the other israised to a
potential Vo. A dielectric slab ofpermittivity
and thickness t (t capacitance of this capacitor.

Answer 1

`C = (\epsilon_0 \epsilon_1 A)/(\epsilon_0 t + \epsilon_1(a-t))\\`

Step-by-step explanation:

If there is a dielectric slab with thickness less than the distance between the plates is inserted inside a capacitor, then that capacitor can be regarded as two capacitors connected in series.

Let's assume that the slab is placed onto the lower side. So, the capacitance of that part of the capacitor, C1 is

`C_1 = \epsilon_1(A)/(t)`

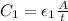
where
`\epsilon_1` is the permittivity of the slab.

The other part of the capacitor is

`C_2 = \epsilon_0 (A)/(a-t)`

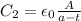
Two capacitors are connected in series:

`(1)/(C) = (1)/(C_1) + (1)/(C_2) = (1)/(\epsilon_1 (A)/(t)) + (1)/(\epsilon_0 (A)/(a-t)) = (t)/(\epsilon_1 A) + (a-t)/(\epsilon_0 A) = (\epsilon_0 t + \epsilon_1 (a-t))/(\epsilon_0 \epsilon_1 A)\\C = (\epsilon_0 \epsilon_1 A)/(\epsilon_0 t + \epsilon_1(a-t))`

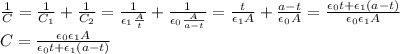
If we know the charge of the plates, we could relate the potential V0 to capacitance via

`C = (Q)/(V_0)`