Question

A 12.0N force with a fixed orientation does work on a

particleas the particle moves through displacement d=(2.00i -4.00j
+3.00k)m. What is the angle between the force and thedisplacement
if the change in the particle's kinetic energy is a)+30.0J and b)
-30.0J ?

Answer 1

(a)
`\theta=62.31^(\circ)`

(b)
`\theta=117.68^(\circ)`

Step-by-step explanation:

It is given that,

Force acting on the particle, F = 12 N

Displacement of the particle,
`d=(2.00i -4.00j+3.00k)\ m`

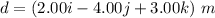
Magnitude of displacement,
`d=√(2^2+4^2+3^2)= 5.38\ m`

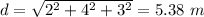
(a) If the change in the kinetic energy of the particle is +30 J. The work done by the particle is given by :

`W=Fd\ cos\theta`

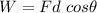
`\theta` is the angle between force and the displacement

According to work energy theorem, the charge in kinetic energy of the particle is equal to the work done.

So,

`cos\theta=(W)/(Fd)`

`cos\theta=(+30\ J)/(12* 5.38)`

`\theta=62.31^(\circ)`

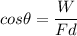
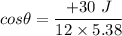
(b) If the change in the kinetic energy of the particle is (-30) J. The work done by the particle is given by :

`cos\theta=(W)/(Fd)`

`cos\theta=(-30\ J)/(12* 5.38)`

`\theta=117.68^(\circ)`

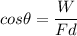
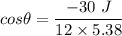
Hence, this is the required solution.