Figure P5.76 is missing from this problem, but I found a similar problem whose figure I uploaded with this answer. The angle of the incline might be different though, but the procedure to follow is the same.
Answer:

Step-by-step explanation:
In order to solve this problem we must first do a drawing of the situation so we can analyze it better. (See attached picture)
Once we have the drawing of the problem, we can go ahead and draw a free body diagram, which will help us determine what forces are acting upon the object. There are different approaches we can take to solve this problem, but I will use an energy balance to do so.
So first we do a sum of forces on the y-axis. This is for us to find what the Normal force is, which will be used to find the force of friction, so we get:

by looking at the free body diagram we get the sum of forces to be:
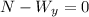
when solving for the normal force we get that:
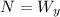
we also know from the free body diagram that:

and
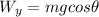
So:
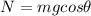
we can also determine the height of the block at the time it is released by analyzing the triangle find in the uploaded figure,, so we get that:

so:
h=21.4cm sin(37°)
h=12.88cm
Once we got this, we can go ahead and do an energy balance on the system, so we get that:

where:
U=potential energy
K=Kinetic energy
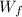 =Work of friction
=Work of friction
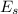 =Potential energy of the spring
=Potential energy of the spring
Since the object is released from rest we know the initial kinetic energy is zero, just like the initial potential energy of the spring since when the block is released, the spring is unstretched. We also know the final potential energy of the block is zero because it reached its lowest point, while the kinetic energy of the block is also zero because it came to rest at that point. This simplifies our energy balance so we get:
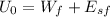
we can now determine each part of the equation so:
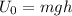
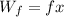
we know that friction is given gy the equation:
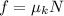
and that
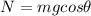
so:
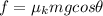
and

and finally:

Once we got all these equations we can substitute them into our balance of energy, so we get:
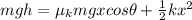
we can now solve this for
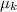 so we get:
so we get:
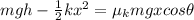
and:
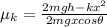
now we can substitute the given data, make sure to use the correct units:
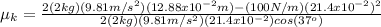
which solves to:
