Question

6. Suppose that a fair coin is tossed 2 times, and the result of each toss (H or T) is recorded.

a. What is the sample space for this chance experiment?
b. For this chance experiment, give the probability distribution for the random variable of the total number of heads observed.

Answer 1

a)
`S= {HH, HT, TH, TT}`

b)
`P(X=0) = (2C0) (0.5)^0 (1-0.5)^(2-0)= 0.25`

`P(X=1) = (2C1) (0.5)^1 (1-0.5)^(2-1)= 0.5`

`P(X=2) = (2C2) (0.5)^2 (1-0.5)^(2-2)= 0.25`

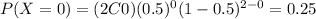
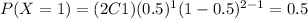
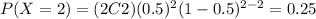
And we have the following table:

X | 0 | 1 | 2

P(X) | 0.25 | 0.5 | 0.25

Explanation:

Let's define first some notation

H= represent a head for the coin tossed

T= represent tails for the coin tossed

We are going to toss a coin 2 times so then the size of the sample size is
`2^2 = 4`

a. What is the sample space for this chance experiment?

The sampling space on this case is given by:

`S= {HH, HT, TH, TT}`

b. For this chance experiment, give the probability distribution for the random variable of the total number of heads observed.

The possible values for the number of heads are X=0,1,2. If we assume a fair coin then the probability of obtain heads is the same probability of obtain tails and we can find the distribution like this:

`P(X=0) = (2C0) (0.5)^0 (1-0.5)^(2-0)= 0.25`

`P(X=1) = (2C1) (0.5)^1 (1-0.5)^(2-1)= 0.5`

`P(X=2) = (2C2) (0.5)^2 (1-0.5)^(2-2)= 0.25`

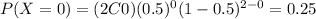
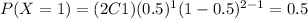
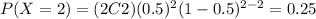
And we have the following table:

X | 0 | 1 | 2

P(X) | 0.25 | 0.5 | 0.25