Question

Thermal Systems, a company that specializes in odor

control,made deposits of $10,000 now, $25,000 at the end of month
6, and$30,000 at the end of month 9. Determine the future worth(end
of year 1) of the deposits at an interest rate of 16% peryear,
compouned quarterly.

Answer 1

$69,939

Explanation:

The equation that describes the future value of an investment with an yearly rate compounded quarterly is:

`FV = PV * (1+(i)/(4))^(4*t)`

Where FV is the future value, PV is hte present value, i is the yearly interest rate, and t is the time in years.

The future value of the deposits is:

`FV = 10,000 * (1+(0.16)/(4))^{4*(12-0)/(12)}+25,000 * (1+(0.16)/(4))^{4*(12-6)/(12)}+30,000 * (1+(0.16)/(4))^{4*(12-9)/(12)}\\FV = \$69,939`

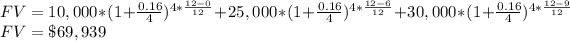
The future worth of the deposits is $69,939.