Question

Mason starts walking from home at 3.7 mph. Ema leaes to follow

48 minutes later, walking at 4.1 mph. How long will it take Ema to
catch up tp Mason ?

Answer 1

It will take Emma 7.4 hours to catch Mason.

Explanation:

Let's build the equations of motion for both Mason and Emma, starting at the minute 48.

These equations have the following format:

`S(t) = S(0) + vt`

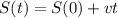
In which S is the position after t minutes, S(0) is the initial position and v is the velocity, in miles per hour.

Mason

Mason walks at 3.7 mph. So
`v = 3.7`.

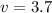48 minutes is 0.8 hour. So at 48 minutes, his position is 0.8*3.7 = 2.96. So S(0) = 2.96.

Mason's equation of motion is:

`S_(M)(t) = 2.96 + 3.7t`

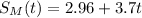
Emma

Emma starts at the position 0, with speed of 4.1mph. o

Emma's equation of motion is:

`S_(E)(t) = 4.1t`

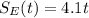
How long will it take Ema to catch up tp Mason ?

This will happen when they are at the same position. So

`S_(M)(t) = S_(E)(t)`

`2.96 + 3.7t = 4.1t`

`0.4t = 2.96`

`t = 7.4`

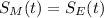
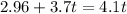
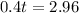
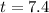
It will take Emma 7.4 hours to catch Mason.