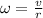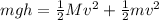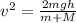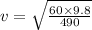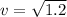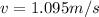Question

If R = 12 cm, M = 430 g, and m = 60 g , find the speed of the block after it has descended 50 cm starting from rest. Solve the problem using energy conservation principles. (Treat the pulley as a uniform disk.)

Answer 1

Step-by-step explanation:

Given

Radius of Pulley r=12 cm

mass of block m=60 gm

mass of Pulley M=430 gm

Block descend h=50 cm

Applying Conservation of Energy

Potential Energy of block convert to rotational Energy of pulley and kinetic energy of block

i.e.

`mgh=(1)/(2)I\omega ^2+(1)/(2)mv^2`

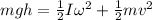
where I=moment of inertia

`I=mr^2`

and for rolling
`\omega =(v)/(r)`

`mgh=(1)/(2)Mv^2+(1)/(2)mv^2`

`v^2=(2mgh)/(m+M)`

`v=\sqrt{(2mgh)/(m+M)}`

`v=\sqrt{(2* 60* 9.8* 0.5)/(430+60)}`

`v=\sqrt{(60* 9.8)/(490)}`

`v=√(1.2)`

`v=1.095 m/s`