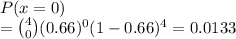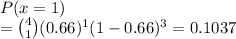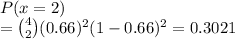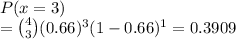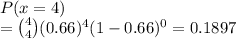Question

In one​ city, the probability that a person will pass his or her driving test on the first attempt is 0.66. Four people are selected at random from among those taking their driving test for the first time. Determine the probability distribution of​ X, the number among the four who pass the test.

Answer 1

P(x = 0) = 0.0133

P(x = 1) = 0.1037

P(x = 2) = 0.3021

P(x = 3) = 0.3909

P(x = 4) = 0.1897

Explanation:

We are given the following information:

We treat person passing the driver test as a success.

P(Adult need eye correction) = 0.66

Then the number of person follows a binomial distribution, where

`P(X=x) = \binom{n}{x}.p^x.(1-p)^(n-x)`

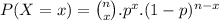
where n is the total number of observations, x is the number of success, p is the probability of success.

Now, we are given n = 4

We have to evaluate the probability distribution function.

`P(x = 0) \\= \binom{4}{0}(0.66)^0(1-0.66)^4 = 0.0133`

`P(x = 1) \\= \binom{4}{1}(0.66)^1(1-0.66)^3 = 0.1037`

`P(x = 2) \\= \binom{4}{2}(0.66)^2(1-0.66)^2 = 0.3021`

`P(x = 3) \\= \binom{4}{3}(0.66)^3(1-0.66)^1 = 0.3909`

`P(x = 4) \\= \binom{4}{4}(0.66)^4(1-0.66)^0 = 0.1897`