Question

Exercise 2

Josie will soon be taking exams in math and Spanish. She estimates that the probability she passes the math exam is 0.9, and the probability that she passes the Spanish exam is 0.8.
She is also willing to assume that the results of the two exams are independent of each other.
a. Using Josie’s assumption of independence, calculate the probability that she passes both exams.
b. Find the probability that Josie passes at least one of the exams. (Hint: Passing at least one of the exams is passing math or passing Spanish.)

Answer 1

a) 0.72

b) 0.98

Explanation:

We are given the following information in the question:

P(passing maths exam) = P(m) = 0.9

P(passing Spanish exam) = P(s) = 0.8

It is assumed that the results of the two exams are independent of each other.

a) probability that she passes both exams

By assumption of independence, we can write:

`P(\text{passes both exam} = P(M\cap S) \\=P(M)* P(S) = 0.8* 0.9 = 0.72`

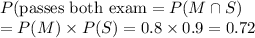
0.72 is the probability that Josie will pass both the exams.

b) probability that Josie passes at least one of the exam

`P(\text{at least one of the exams}) = P(M\cup S)\\= P(M) + P(S) - P(M\cap S)\\= 0.9 + 0.8 - 0.72 = 0.98`

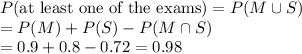
0.88 is the probability that Josie passes at least one of the exams.