Question

Divide. HELP ASAP PLEASE!!

Answer 1

The answer to your question is below

Explanation:

a)
`(s^(2)- 3s - 28 )/(s - 7)`

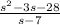
Factor the numerator is a polynomial of the form x² + bx + c

s² - 3s - 28 = (s - 7)( s + 4)

Substitute

`((s - 7)(s + 4))/((s - 7))`

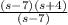
Simplify like terms

Result s + 4

b)
`(c^(2) + 5c - 24)/(c + 8)`

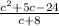
Factor the numerator is a polynomial of the form x² + bx + c

c² + 5c - 24 = (c + 8)(c - 3)

Substitute

`((c + 8)(c - 3))/(c + 8)`

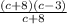
Simplify like terms c - 3

Result c - 3