Question

Find the remaining sides of a 30, 60, 90 degree triangle if the side opposite of 60 degrees is 4.

Answer 1

The remaining sides are
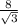`(8)/(√(3))` units and
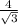`(4)/(√(3))` units.

Explanation:

It is given that the interior angles of a right angle triangle are 30, 60, 90 degree. The side opposite of 60 degrees is 4.

In a right angle triangle,

`\sin \theta = (opposite)/(hypotenuse)`

`\sin (60) = (4)/(hypotenuse)`

`(√(3))/(2) = (4)/(hypotenuse)`

`hypotenuse =4* (2)/(√(3))`

`hypotenuse =(8)/(√(3))`

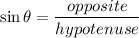
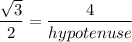
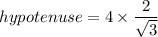
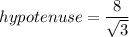
The hypotenuse of the triangle is
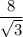`(8)/(√(3))` units.

According to the Pythagoras theorem,

`hypotenuse^2 =perpendicular^2+base^2`

`((8)/(√(3)))^2 =(4)^2+base^2`

`(64)/(3) =16+base^2`

`(64)/(3)-16=base^2`

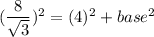
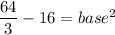
Taking square root on both sides.

`\sqrt{(64-48)/(3)}=base`

`\sqrt{(16)/(3)}=base`

`(4)/(√(3)) =base`

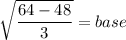
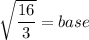
Therefore, the remaining sides are
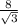`(8)/(√(3))` units and
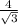`(4)/(√(3))` units.