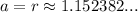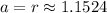Answer:
r ≈ 1.1524
Explanation:
The radius ( r ) of the circle circumscribed can be obtained using the Law of cosines, and seven of them can be traced from circle's center to each vertex of the heptagon, forming seven identical triangles.
For each triangle, c (one of the sides of the heptagon) is the opposite side to the angle
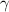 with known value (c = 1), and sides a and b have the same size of the radius of the circle we are about to find (a = b = r).
with known value (c = 1), and sides a and b have the same size of the radius of the circle we are about to find (a = b = r).
The Law of cosines states that:
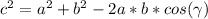 [1]
[1]
Since we have c, and we know the angle
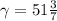 , i.e., the total of degrees of the circle divided by 7, a = b (for the reason already explained), then, we have all elements to solve the equation [1] for the radius of the circle (a = b = r):
, i.e., the total of degrees of the circle divided by 7, a = b (for the reason already explained), then, we have all elements to solve the equation [1] for the radius of the circle (a = b = r):
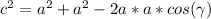
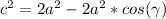
![\\ c^2 = 2a^2 [ 1 - cos(\gamma) ]](https://img.qammunity.org/2021/formulas/mathematics/college/s7zh533c4uj8xynzol14vgi4vy5hkh89c1.png)
For c = 1
![\\ 1^2 = 2a^2 [ 1 - cos(\gamma) ]](https://img.qammunity.org/2021/formulas/mathematics/college/ttngvj06zqhklxc8mxx3uukhe0rphpyz9u.png)
![\\ 1 = 2a^2 [ 1 - cos(\gamma) ]](https://img.qammunity.org/2021/formulas/mathematics/college/y2smwll9u34xibqlxqh8koh5ojwvj50848.png)
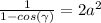
![\\ (1)/(2[1 - cos(\gamma)]) = a^2](https://img.qammunity.org/2021/formulas/mathematics/college/vbvo8770par7k6jpdmybs27mg3sejsvipm.png)
![\\ a = \sqrt(1)/(2[1 - cos(\gamma)])](https://img.qammunity.org/2021/formulas/mathematics/college/a5flpbwrldk9i9sd7k6au3o1rgmt27v3di.png)
Substituting
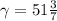
![\\ a = \sqrt(1)/(2[1 - cos(51(3)/(7))])](https://img.qammunity.org/2021/formulas/mathematics/college/omhervaacu5ceqsc0ybezcmldnw2cz254f.png)
Thus, the radius of the circle circumscribed about the heptagon is: