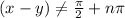Question

Show tan(???? − ????) = tan(????)−tan(????) / 1+tan(????) tan(????)
.

Answer 1

See the proof below

Explanation:

For this case we need to proof the following identity:

`tan(x-y) = (tan(x) -tan(y))/(1+ tan(x) tan(y))`

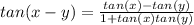
We need to begin with the definition of tangent:

`tan (x) =(sin(x))/(cos(x))`

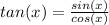
So we can replace into our formula and we got:

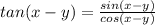`tan(x-y) = (sin(x-y))/(cos(x-y))` (1)

We have the following identities useful for this case:

`sin(a-b) = sin(a) cos(b) - sin(b) cos(a)`

`cos(a-b) = cos(a) cos(b) + sin (a) sin(b)`

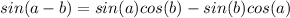
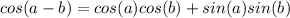
If we apply the identities into our equation (1) we got:

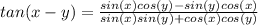`tan(x-y) = (sin(x) cos(y) - sin(y) cos(x))/(sin(x) sin(y) + cos(x) cos(y))` (2)

Now we can divide the numerator and denominato from expression (2) by
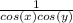`(1)/(cos(x) cos(y))` and we got this:

`tan(x-y) = ((sin(x) cos(y))/(cos(x) cos(y)) - (sin(y) cos(x))/(cos(x) cos(y)))/((sin(x) sin(y))/(cos(x) cos(y)) +(cos(x) cos(y))/(cos(x) cos(y)))`

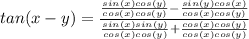
And simplifying we got:

`tan(x-y) = (tan(x) -tan(y))/(1+ tan(x) tan(y))`

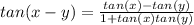
And this identity is satisfied for all:

`(x-y) \\eq (\pi)/(2) +n\pi`