Question

Sara and Paul are on opposite sides of a building that a telephone pole fell on. The pole is leaning away from Paul at

an angle of 59° and towards Sara. Sara measures the angle of elevation to the top of the telephone pole to be 22°,
and Paul measures the angle of elevation to be 34°. Knowing that the telephone pole is about 35 ft. tall, answer the
following questions.
a. Draw a diagram of the situation.
b. How far apart are Sara and Paul?
c. If we assume the building is still standing, how tall is the building?

Answer 1

a) See figure attached

b)
`x = (sin(124))/(sin(34)) 80.086 = 118.732 ft`

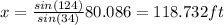
c)
`h = 35 sin (59) = 30.0 ft`

So then the heigth for the building is approximately 30 ft

Explanation:

Part a

We can see the figure attached is a illustration for the problem on this case.

Part b

For this case we can use the sin law to find the value of r first like this:

`(sin(22))/(35 ft) =(sin(59))/(r)`

`r= (sin(59))/(sin(22)) 35 ft = 80.086ft`

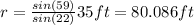
Then we can use the same law in order to find the valueof x liek this:

`(sin(124))/(x ft) =(sin(34))/(80.086)`

`x = (sin(124))/(sin(34)) 80.086 = 118.732 ft`

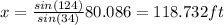
And that represent the distance between Sara and Paul.

Part c

For this cas we are interested on the height h on the figure attached. We can use the sine indentity in order to find it.

`sin (59) = (h)/(35)`

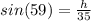
And if we solve for h we got:

`h = 35 sin (59) = 30.0 ft`

So then the heigth for the building is approximately 30 ft