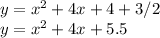Answer:
a.
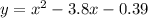
b.
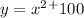
c.
Explanation:
Many different graphics can have the same vertex.
The equation of a parabola in the form of a vertex is:

where
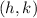 is the vertex
is the vertex
- for a.
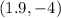
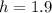
and
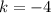
so the equation is:
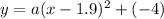
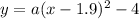
As we are not given the value of
 , and we are only asked for an equation we can choose the value we like. I will choose a = 1 to simplify
, and we are only asked for an equation we can choose the value we like. I will choose a = 1 to simplify
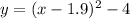
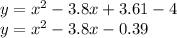
- for b.
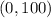
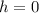
and
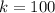
so the equation is:
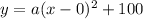
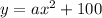
again choosing a=1
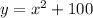
- for c.
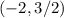
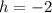
and
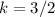
so the equation is:
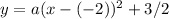
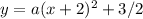
again choosing a=1