Question

Use the given information to find the amount in the account earning compound interest after six years with the principle is 3500 R equals 2.29% compounded monthly

Answer 1

To find the amount in the account earning compound interest after six years with a principal of 3500 R and an interest rate of 2.29% compounded monthly, you can use the compound interest formula.

Step-by-step explanation:

To find the amount in the account earning compound interest after six years with a principal of 3500 R and an interest rate of 2.29% compounded monthly, we can use the compound interest formula.

1. Convert the interest rate to a decimal by dividing it by 100: 2.29 / 100 = 0.0229.
2. Divide the interest rate by the number of compounding periods per year to get the monthly interest rate: 0.0229 / 12 = 0.0019083.
3. Multiply the principal by (1 + the monthly interest rate) raised to the power of the number of compounding periods (6 years * 12 months per year): 3500 * (1 + 0.0019083)^72 = 3500 * 1.15085 ≈ 4029.48 R.

The amount in the account earning compound interest after six years would be approximately 4029.48 R.

Answer 2

The Amount in account after 6 years is $4014.976

Step-by-step explanation:

Given as :

The principal = p = $3500

The rate of interest = r = 2.29% compounded monthly

The time period = t = 6 years

Let The Amount in account after 6 years = $A

From Compound Interest method

Amount = Principal ×
`(1+(\textrm rate)/(12* 100))^(12* time)`

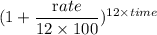
Or, A = p ×
`(1+(\textrm r)/(12* 100))^(12* t)`

Or, A = $3500 ×
`(1+(\textrm 2.29)/(12* 100))^(12* 6)`

Or, A = $3500 ×
`(1.0019083)^(72)`

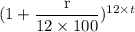
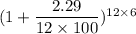
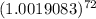
Or, A = $3500 × 1.147136

∴ A = $4014.976

So,The Amount in account after 6 years = A = $4014.976

Hence, The Amount in account after 6 years is $4014.976 Answer