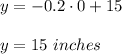Question

A candlestick burns at a rate of 0.2 inches per hour. After eight straight hours of burning, the candlestick is 13.4 inches tall. Write and solve a linear equation to find the original height of the candle. Write using point & slope applications

Answer 1

`y=-0.2x+15\\ \\15\ inches`

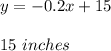
Explanation:

Let

x = time (number of hours the cande was burning)

y = height of the candle after x hours of burning

A candlestick burns at a rate of 0.2 inches per hour. This means the slope of the linear function is
`m=-0.2`

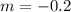
The equation of the linear function is

`y=-0.2x+b`

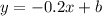
After 8 straight hours of burning, the candlestick is 13.4 inches tall. This means x = 8 and y = 13.4, then

`13.4=-0.2\cdot 8+b\\ \\b-1.6=13.4\\ \\b=13.4+1.6\\ \\b=15`

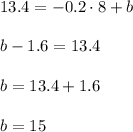
Hence, the equation of the linear function is

`y=-0.2x+15`

Initially, at x = 0, the height of the candle was

`y=-0.2\cdot 0+15\\ \\y=15\ inches`