Question

A Square Pyramid has a Total Surface Area of 40

square inches and the side length of its square base
is 4 inches. Determine the slant height of the
pyramid.​

Answer 1

Explanation:

1. 9 square inches

2. 60 square inches

3. 69 square inches

Answer 2

`3\ in`

Explanation:

Let h be the slant height of the square pyramid.

Given:

Total surface area of the square pyramid = 40 square inches

And square base has a side length = 4 inches

We need to find slant height of the square pyramid.

The surface area of the pyramid is sum of the area of the square and area of each of the triangle faces.

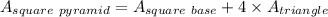`A_(square\ pyramid) = A_(square\ base) + 4* A_(triangle)`-------(1)

The base area of the square pyramid =
`(side)^(2)`

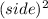
So,
`A_(square\ base) = 4^(2)`

`A_(square\ base) = 16  in^(2)`

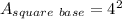
And area of the triangle =
`(1)/(2) b* h`

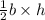
Where b = base of the triangle

The base of the triangle is one side of the square pyramid base, so the base of the triangle is 4 inches.

And h = slant height of the triangle.

`A_(triangle) = (1)/(2)* 4* h`

`A_(triangle) = 2* h`

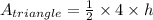
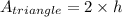
So the area of the triangle = 2h square inches

Now we substitute area of the base and area of the triangle in equation 1.

`A_(square\ pyramid) = 16 + 4* 2h`

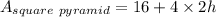
Now we substitute the value of total surface area of the square pyramid in above equation.

`40 = 16 + 4* 2h`

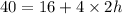
Simplify the above equation.

`40 = 16 + 8h`

`8h = 40-16`

`8h = 24`

`h = (24)/(8)`

`h=3\ in`

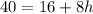
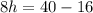
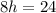
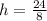
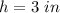
Therefore the slant height of the square pyramid is 3 inches.