Question

Printer A prints 36 pages every 1.5 minutes. Printer b prints 114 pages every 3 minutes printer c prints 115 pages every 5 minutes which printer prints the fastest?

Answer 1

printer C

Explanation:

because if you divide it it will round up

Answer 2

Printer B prints faster

Solution:

Given that Printer A prints 36 pages every 1.5 minutes

Let "x" be the number of pages printed in 1 minute

Therefore,

1.5 minutes = 36 pages

1 minute = x pages

By cross-multiplication,

`1.5 * x = 36 * 1\\\\x = (36)/(1.5) = 24`

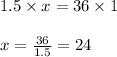
Thus unit rate of Printer A is: In 1 minute, Printer A can print 24 pages

Printer B prints 114 pages every 3 minutes

Similarly,

3 minutes = 114 pages

1 minute = x pages

This forms a proportion. Therefore by crossmultiplying we get,

`3 * x = 114 * 1\\\\x = (114)/(3) = 38`

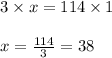
Thus unit rate of Printer B is: In 1 minute, Printer B can print 38 pages

Printer C prints 115 pages every 5 minutes

Similarly,

5 minutes = 115 pages

1 minute = x pages

This forms a proportion. Therefore by crossmultiplying we get,

`5 * x = 1 * 115\\\\x = (115)/(5) = 23`

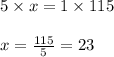
Thus unit rate of Printer C is: In 1 minute, Printer C can print 23 pages

unit rate of printer B > unit rate of printer A > unit rate of printer C

On comparing the unit rate of Printer A, B, C we see that, printer B prints faster