Answer:
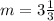
Explanation:
Given,
△ART∼△EFG
And also given that EG = GF
We have to find the measure of 'm'.
Solution,
Since △ART∼△EFG
So according to the property of similarity, which states that;
"If two triangles are similar then the ratio of corresponding sides are equal".

And EG = GF,
So we can say that;
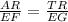
Now putting the given values, we get;
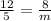
On using cross multiplication method, we get;
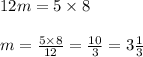
Hence the value of 'm' is
 .
.