Question

Driving times for students' commute to school is normally distributed, with a mean time of 14 minutes and a standard deviation of 3 minutes. Using the empirical

rule, approximately what percent of students' commute time is between 11 and 17 minutes?
32%
68%
95%
99.7%

Answer 1

Using the empirical rule, approximately 68 percent of students' commute time is between 11 and 17 minutes

Explanation:

Given that driving times for students' commute to school is normally distributed, with a mean time of 14 minutes and a standard deviation of 3 minutes. So, first we have to find z-score of 11 and 17 using z-score formula.

`z=(x-\mu)/(\sigma)=(11-14)/(3)=(-3)/(3)=-1`

`z=(17-14)/(3)=(3)/(3)=1`

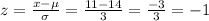
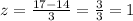
Also, we do aware that z-score says us a data point is how many standard deviations above or below mean. This z-score -1 and 1 indicates that 11 and 17 lie within one standard deviation of the mean. Therefore, by empirical rule, 68% data lies within one standard deviation of the mean. So, Option B is correct.