Question

If the perimeter of the large square tile is 48 inches and the perimeter of the smaller square is 16 inches, what is the perimeter of one of the trapezoids? A 19.7 inches B 25.4 inches C 27.3 inches D 32 inches

Answer 1

The perimeter of one of the trapezoids is equal to 27.3 inches

Explanation:

Given:

Perimeter of the large square tile = 48 inches

Perimeter of the smaller square = 16 inches

To Find :

The perimeter of one of the trapezoids

see the attached figure to better understand the problem

Solution:

we know that

The perimeter of a square is

P =
`4 * sides`

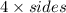
step 1:Find the length side of the smaller square

`16=4 * side_(\small square)`

`side_(\small square)= (16)/(4)`

`side_(\small square)= 4 inches`

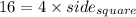
step 2 :Find the length side of the large square

`48=4 * side _(large square)`

`side _(large square)=(48)/(4)`

`side _(large square)=12 inches`

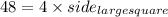
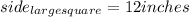
step 3: Find the height of one trapezoid

The height is equal to

`h=((12-4))/(2)`=4 in

step 4: Find the hypotenuse of one isosceles right triangle

one trapezoid is equal to one square and two isosceles right triangles.

Applying Pythagoras Theorem

`c^(2)=4^(2) +4^(2) \\ \\c=4√(2)\ in`

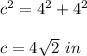
step 5: Find the perimeter of one of the trapezoid

The perimeter is equal to

`P=(4√(2) +4+4√(2)+12)\\ \\P=(16+8√(2))\ in`

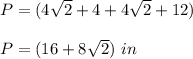
P = 16 +8(1.414)

P= 16 + 11.28

P= 27.28

P =27.3