Question

A meter stick is held vertically with one end on the floor and is then allowed to fall. Find the speed of the other end when it hits the floor, assuming that the end on the floor does not slip. (Hint: Consider the stick to be a thin rod and use the conservation of energy principle.)

Answer 1

5.4 ms⁻¹

Step-by-step explanation:

Here we have to use conservation of energy. Initially when the stick is held vertical, its center of mass is at some height above the ground, hence the stick has some gravitational potential energy. As the stick is allowed to fall, its rotates about one. gravitational potential energy of the stick gets converted into rotational kinetic energy.

`L` = length of the meter stick = 1 m

`m` = mass of the meter stick

`w` = angular speed of the meter stick as it hits the floor

`v` = speed of the other end of the stick

we know that, linear speed and angular speed are related as

`v = r w\\w = (v)/(r)`

`h` = height of center of mass of meter stick above the floor =
`(L)/(2) = (1)/(2) = 0.5 m`

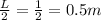
`I` = Moment of inertia of the stick about one end

For a stick, momentof inertia about one end has the formula as

`I = (mL^(2) )/(3)`

Using conservation of energy

Rotational kinetic energy of the stick = gravitational potential energy

`(0.5) I w^(2) = mgh\\(0.5)((mL^(2) )/(3)) ((v)/(L) )^(2) = mgh\\(0.5)((v^(2) )/(3)) = gh\\(0.5)((v^(2) )/(3)) = (9.8)(0.5)\\v = 5.4 ms^(-1)`